

I bought a board game with four different colored sets of polyiamonds. The pieces have one up to six triangles and each set consists of 22 two-sided pieces. Instead of playing the game I tried to make symmetric constructions with these sets so that same colored pieces don't touch at their edges. This way the shapes of the pieces are separated and it's easier to spot them in a construction.
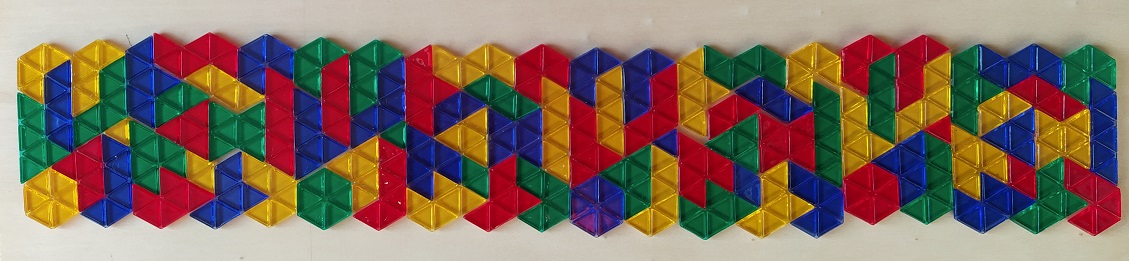
Furthermore I looked at sets of polyiamonds with more than six triangles for each piece. If pieces with less than five triangles are missing in a set or if only three colors are given, the construction are more difficult and a computer program is useful. Sometimes a solution for a third or fourth of a construction is possible and can be extended by a colorshift for the remaining parts. This also applies for hand solving as the following picture shows.
The following table shows some sets of pieces with a fixed number of triangles. They can be used for constructions like parallelograms, hexagons, hexagonal rings or stars with some kind of symmetry. Click the numbers of pieces to see one or more figures.
| Basic Sets | Number of squares | Property | One Set | Three Sets | Four Sets | |||
|---|---|---|---|---|---|---|---|---|
| Number of Pieces | Total Area | Number of Pieces | Total Area | Number of Pieces | Total Area | |||
| Tetriamonds | 4 | Two-sided | 3 | 12 | 9 | 36 | 12 | 48 |
| One-sided | 4 | 16 | 12 | 48 | 16 | 64 | ||
| Pentiamonds | 5 | Two-sided | 4 | 20 | 12 | 60 | 16 | 80 |
| One-sided | 6 | 30 | 18 | 90 | 24 | 120 | ||
| Hexiamonds | 6 | Two-sided | 12 | 72 | 36 | 216 | 48 | 288 |
| One-sided | 19 | 114 | 57 | 342 | 76 | 456 | ||
| Heptiamonds | 7 | Two-sided | 24 | 168 | 72 | 504 | 96 | 672 |
| One-sided | 43 | 301 | 129 | 903 | 172 | 1204 | ||
| Octiamonds | 8 | Two-sided | 66 | 528 | 198 | 1584 | 264 | 2112 |
| One-sided | 120 | 960 | 360 | 2880 | 480 | 3840 | ||

In the pictures you can see pieces with different numbers of triangles. This avoids problems with an odd number of unbalanced pieces and gives the chance to keep back smaller pieces for the end of a construction. Only one or few examples for combined sets are shown. Click the numbers of pieces to see the figures.
| Basic Sets | Number of squares | Property | One Set | Three Sets | Four Sets | |||
|---|---|---|---|---|---|---|---|---|
| Number of Pieces | Total Area | Number of Pieces | Total Area | Number of Pieces | Total Area | |||
| Polyiamonds of Order 1 to 6 | 1..6 | Two-sided | 22 | 110 | 66 | 330 | 88 | 440 |
| One-sided | 30 | 166 | 90 | 498 | 120 | 664 | ||
| Tetriamonds ∪ Pentiamodns ∪ Hexiamonds | 4..6 | Two-sided | 19 | 104 | 57 | 312 | 76 | 416 |
| One-sided | 27 | 160 | 81 | 480 | 108 | 640 | ||
| Pentiamonds ∪ Hexiamonds | 5 or 6 | Two-sided | 16 | 92 | 48 | 276 | 64 | 368 |
| One-sided | 23 | 144 | 69 | 432 | 92 | 576 | ||
| Hexiamonds ∪ Heptiamonds | 6 or 7 | Two-sided | 36 | 240 | 108 | 720 | 144 | 960 |
| One-sided | 62 | 415 | 186 | 1245 | 248 | 1660 | ||
