107 Hexaboloes
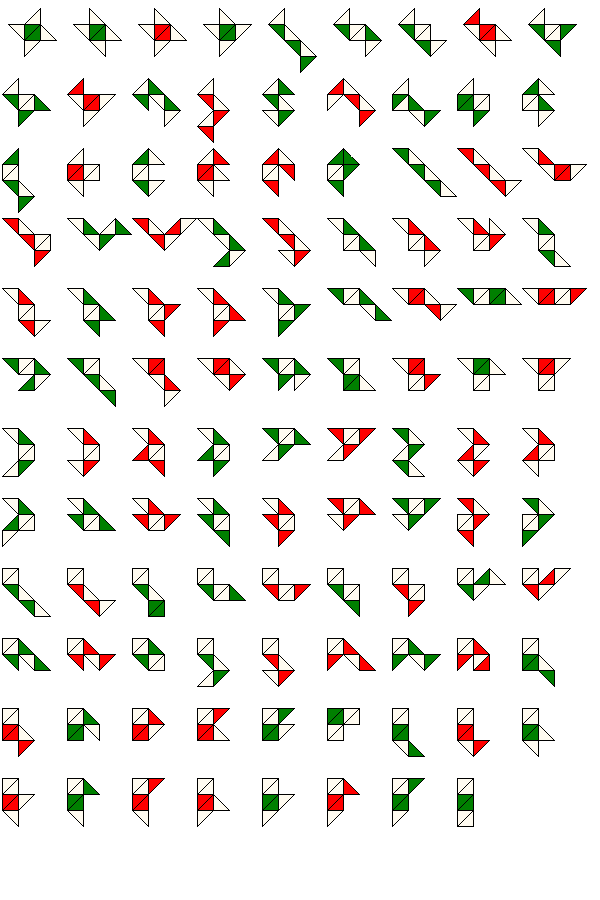
The picture shows the 107 hexaboloes. The green pieces
have even numbers of parallel h-edges, the red ones odd numbers.
Since there are 58 green pieces and 49 red pieces the total numbers
of parallel h-edges must be odd. As in the case of tetraboloes a
rectangle with all hexaboloes is impossible to construct. Each pattern symmetric under
180 degree rotation has even numbers of up and down h-edges and therefore can't
be build with all hexaboloes. Is there only one axis for reflection complete
constructions are possible.
There is another constraint due to a checkering argument. If you count the triangles which cover
black squares of a checker board you find balanced pieces with three black and three white triangles
and "even" pieces with an even number of black and white triangles. Since the total number of
balanced pieces is odd all constructions with the whole set must cover an odd number of black
triangles.
index -
previous -
next

