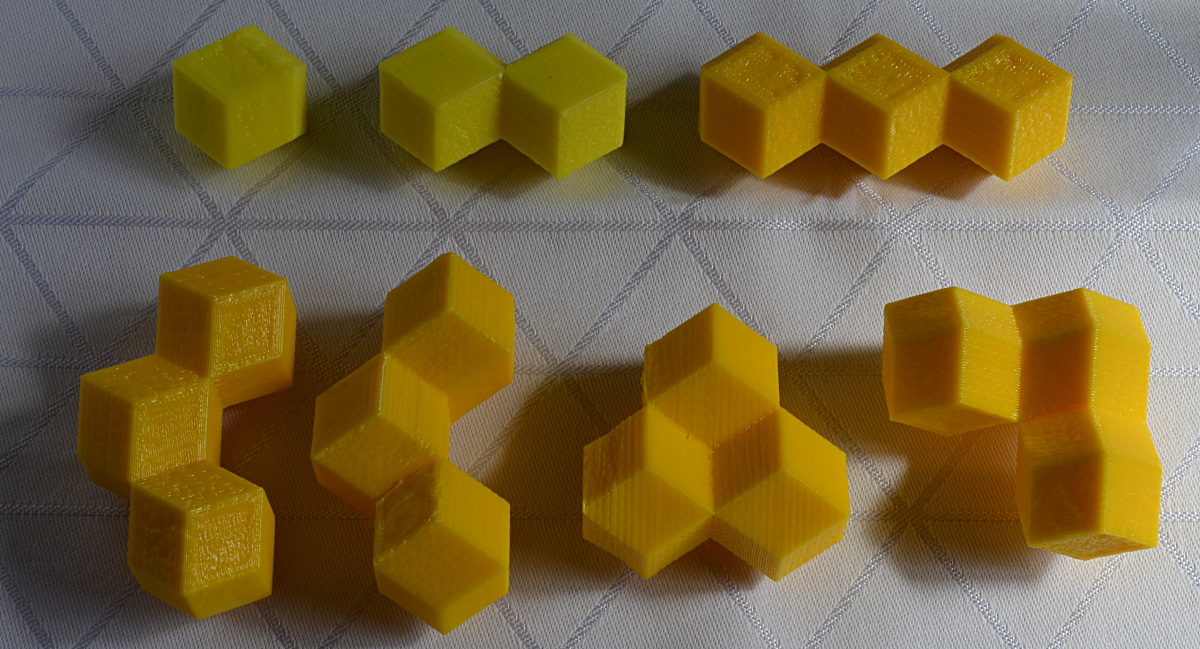
Polyrhons of Order 1 - 3
There are only seven polyrhons composed of three or less rhombic dodecahedrons and
we get a total number of 18 units.
We can try to make a kind of platform with 3x4 elements in the bottom layer and 2x3 elements in the top layer.
Depending on the orientation of the elements we get three diferent problems. It's easy to solve them
without help of a computer.

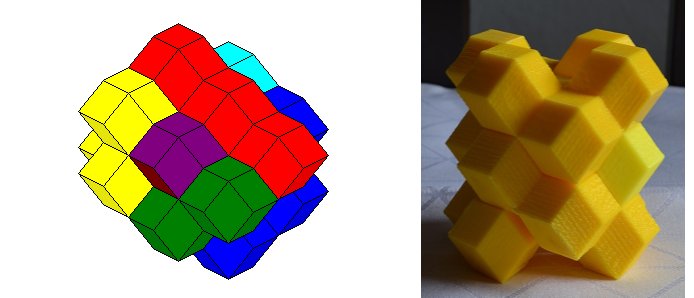
To get constructions with box symmetry we can combine two layers of size 2x3 for the bottom and the top layer and one
layer of size 3x2 between them. For short I labeled this construction as 2(2x3)+1(3x2).
If the construction is turned around so that rhombic faces are at the bottom you can get
a stable physical puzzle.
A figure with three 2x2 layers and two 1x3 layers has also box symmetry
but must also be turned around to get a stable physical construction

A tetrahedron of edge length 2 plus a square pyramid with edge length 3 is possible, too.

At last a figure with triangle symmetry is shown.

Back
Home






