5 Squares + Tan, 210 Two-sided Pieces
Given a total area of 1155 unit squares we can construct 15 rectangles of size 11x7. These rectangles can be combined to get larger ones.

Symmetric convex octagons are also possible. You can start with a 51x23 rectangle and cut off four triangles of size 3 at the corners.

Four tans removed at the corners of a 89x13 rectangle is another option.

A symmetric hexagon is given by removing single tans at two corners of a 34x34 square.

A 39x31 rectangle with 54 holes has also two axes of symmetry.

5 Squares + Tan, 417 One-sided Pieces
Since 217=20*20+4*4+1 it should be possible to construct 20-fold and
4-fold replicas of pieces which don't have sharp angles. First you must create the long diagonal edge, then the 4-fold replica, and at last five stripes can finish the 20-fold replica. Two examples are shown.


Since the total area of 2293.5 unit squares isn't an integer rectangles can't be constructed. Instead I made a 106x23 rectangle with one corner cut off by a triangle of size 17 getting a convex pentagon.

An octagon with one axis of symmetry is also possible if you take a 49x49 square and cut off two triangle of size 3 and two triangles of size 14 and 1.

Pentominoes + Tan, 171 Two-sided Pieces
Total area is 940.5 unit squares. Therefore rectangles are impossible.
Here is a 35x27 rectangle with one triangle of size 3 cut off.
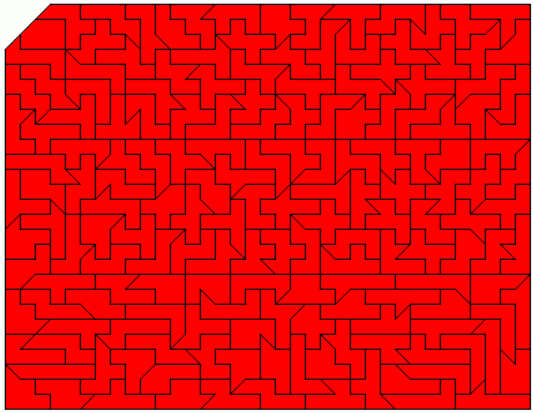
You get a symmetric convex heptagon if you take a 34x34 square and cut off two triangles of size 4 and one triangle of size 3.
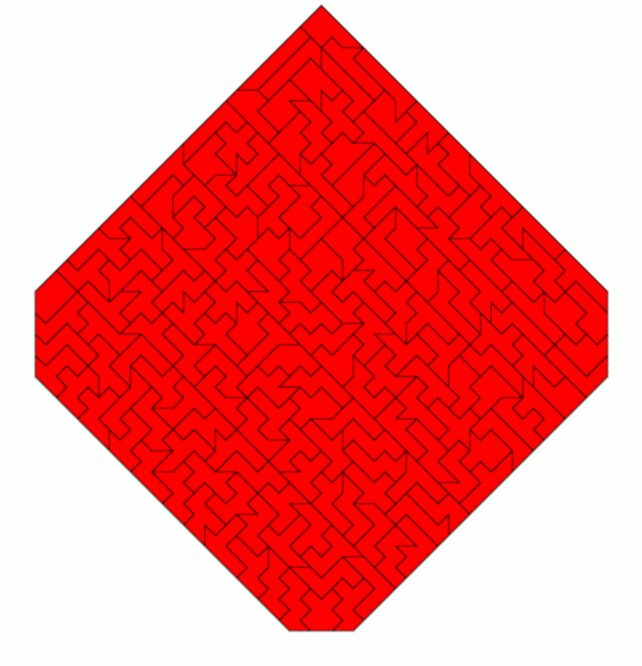
Pentominoes + Tan, 339 One-sided Pieces
Total area is 1864.5 unit squares, not suitable for rectangles. I tried to get a sliced rectangle with a long diagonal edge. Here is a 49x41 rectangle with a triangle of size 17 cut off.

Two convex symmetric heptagons are derived from 45x45 squares. The first one is given by cutting off triangles of size 11, 10, 11.

For the second one triangles of size 14, 17, 14 are cut off.

Hexominoes - Tan, No 315° Angles, 175 Two-sided Pieces
Total area is 962.5 unit squares, rectangles are impossible.
I took a 33x31 rectangle and cut off a triangle of size 11 to get a convex pentagon.

The convex symmetric heptagon shown below is a 32x32 square where triangles of size 7, 5, 7 are cut off.
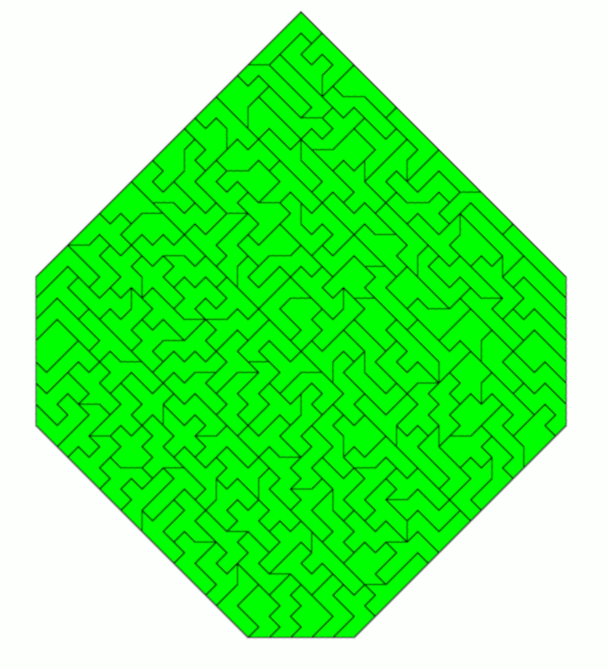
Hexominoes - Tan, No 315° Angles, 347 One-sided Pieces
Total area is 1908.5 unit squares. An example for a sliced rectangle is a 47x43 rectangle with a triangle of size 15 removed.

The convex symmetric heptagon shown below is a 45x45 square where triangles of size 2, 15, 2 are cut off.
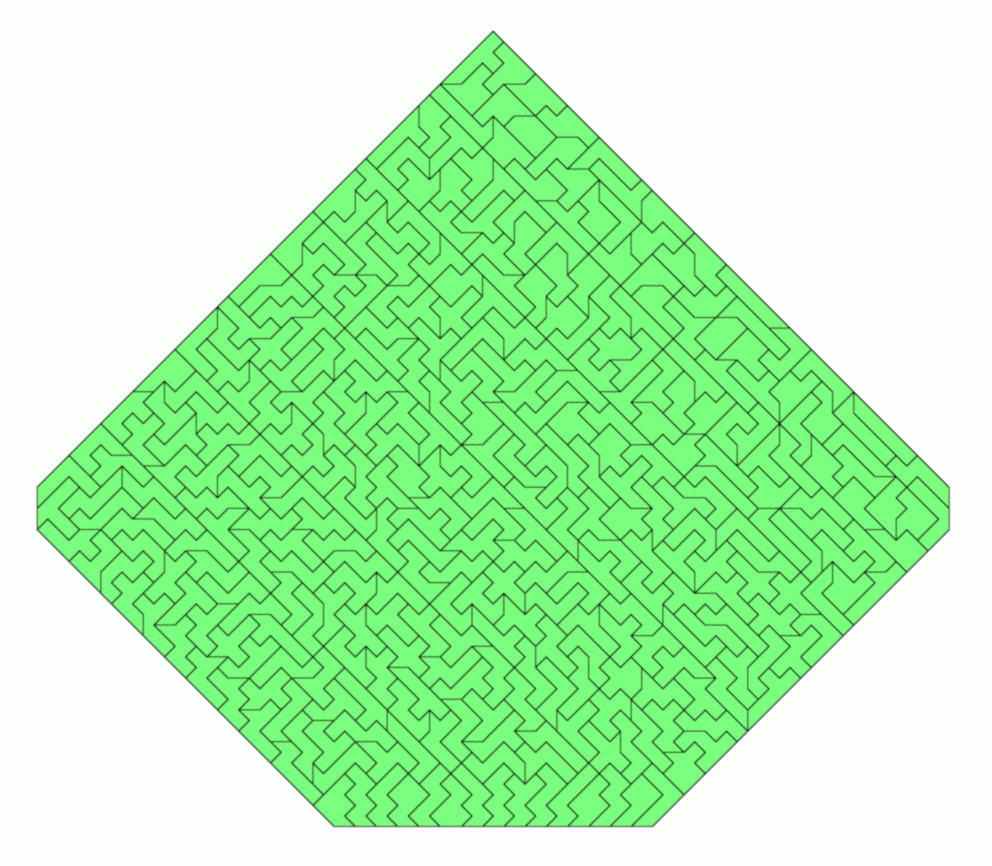
Pentominoes + Tan, No 315° Angles, 143 Two-sided Pieces
Total area is 786.5 unit squares. Examples for sliced rectangles are a 49x19 rectangle with a triangle of size 17 removed and a 31x29 rectangle with a triangle of size 15 removed.

The convex symmetric heptagon shown below is a 30x30 square where triangles of size 1, 15, 1 are cut off.

Pentominoes + Tan, No 315° Angles, 283 One-sided Pieces
Total area is 1556.5 unit squares. An example for a sliced rectangle is a 63x27 rectangle with a triangle of size 17 removed.

The convex symmetric heptagon is a 41x41 square where triangles of size 8, 11, 8 are cut off.

![]()
Back
Home






















