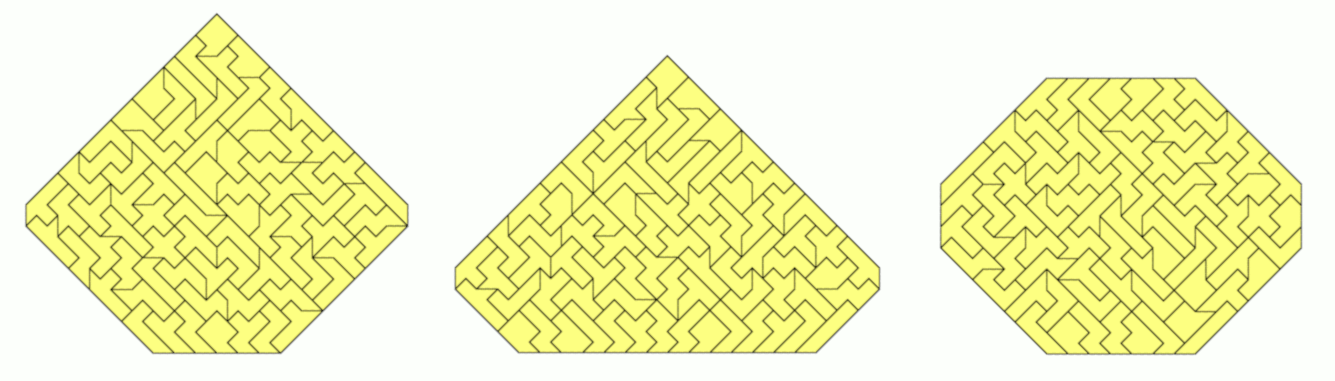
The total area of all pieces is 243=3*9*9. Therfore I looked for three copies of symmetric figures. Here are three jagged squares with symmetries like usual squares.
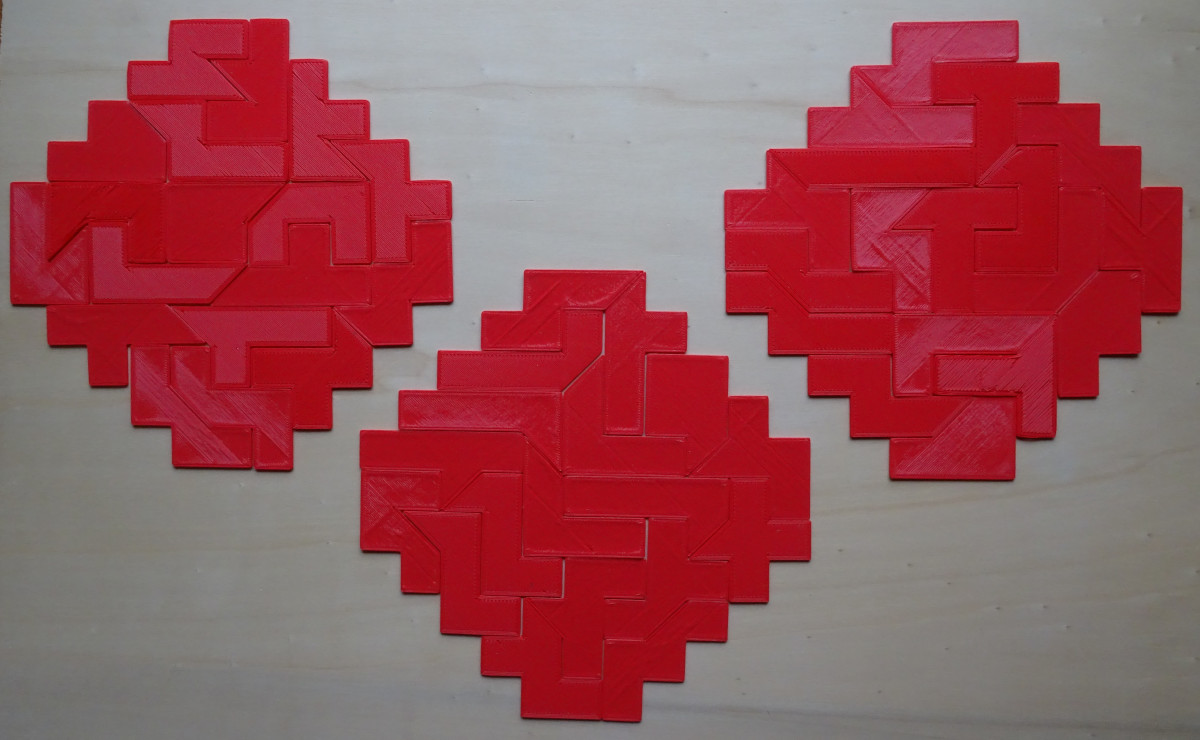
Beside the known 9x9 squares further 3-fold construction are possible.

You get symmetric octagons if rectangles of size 49x5 or 35x7 are sliced at each corner by one tan. I added a construction of an octagon by removing triangles of size 2 and 3 from a 16x16 square also with two axes of symmetry.
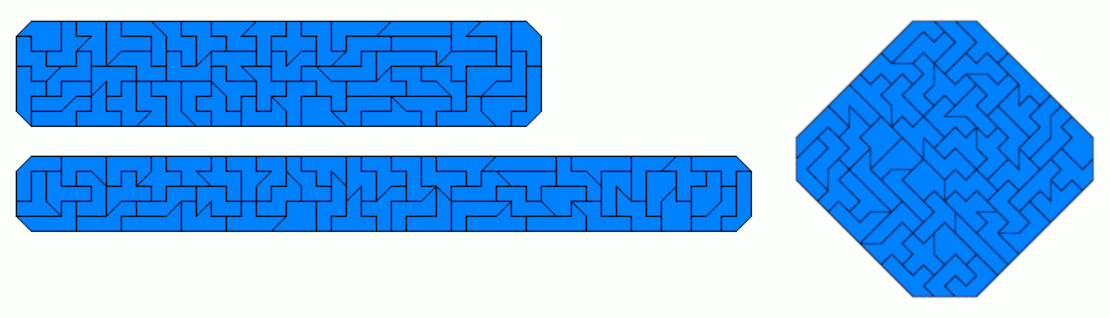
You can also cut off one, two or three corners to get a convex pentagon, hexagon or heptagon.

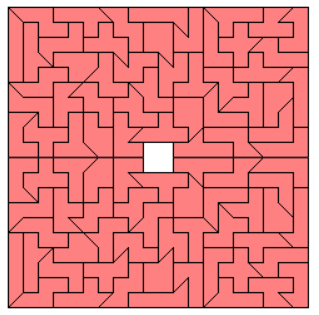
At last I tried to construct a rectangle with symmetrically placed holes.


Beside the known sliced rectangles with slices of length 4 and 8 you can even take 17x31 or 23x25 rectangles and remove triangles of size 10 or 14, respectively.

You can get octagons with two axes of symmetry by slicing all corners of 45x11 or 97x5 rectangles with equal cuts of size 3 or 2, respectively. Starting with a 23x23 square and cuts of size 4 and 6 another octagon with two axes of symmetry is possible.


A lot of pentagons is already know. As convex, symmetric polygons I added a hexagon and a heptagon.

Beside octagons with sliced corner of size 1 you can also get an octagon with sliced corners of size 3 from a 18x12 rectangle.


A symmetric hexagon and an octagon can also be constructed. We start with a 20x20 or 21x21 squares and cut triangles of size 2 or 3 and 6, respectively. By reflecting a pentagon or a hexagon made with the two-sided pieces a different hexagon and an octagon are possible.

Two heptagons cut from 67x6 and 57x7 rectangles are further examples of convex polygons. The pentagon at the right, cut from a 23x19 rectangle, can also be changed to another one with height 9.

Using the mirror method a ring with square symmetry is made.

I also looked for other polygons. A hexagon cut from a 22x10 rectangle, a symmetric heptagon cut from a 15x15 square and a symmetric octagon cut from a 15x15 square are shown.


Pentagons with sliced edges of size 2 or 4 or known and I tried to get larger slices. Slices of size 10, 12 or 14 are possible cutting rectangles of size 29x16, 27x18 or 32x26, respectively.

Cutting rectangles of size 43x19, 21x21 or 52x8 we can get a symmetric hexagon, a symmetric heptagon or a symmetric octagon.


We can remove triangles of size 6 and 10 from rectangles of size 27x7, 21x9 and 19x13 to get pentagons.

A symmetric hexagon and two symmetric octagons are cut from 14x14 or 21x9 rectangles. The heptagon isn't symmetric.


Some pentagons with sliced edges of size 12, 14 an 18 are shown. They are derived from rectangles of size 23x18, 22x20 and 24x21, respectively.

An example of a symmetric hexagon is a 49x7 rectangle with single tans cut off at two corners. Other hexagons are given by a pentagon made from two-sided pieces and its mirror figure. The octagon is a 43x8 rectangle without single tans at all corners.

The left heptagon is a 19x19 square, where triangles of size 1, 6 and 1 are cut off. The second heptagon is a 21x21 square, where triangles of size 1, 14, and 1 are cut off. The octagon at the right has sliced edges of size 3, 7, 3 and 7 giving a total of 20 sliced squares at the edges. A 20x20 square was cut off.