Notched S-Tetrominoes

It's impossible to fill a rectangle with S-tetrominoes. Therefore I decided to join sets of notched S-tetrominoes to sets of other tetrominoes with the same number of notches. This way some rectangles can be constructed. The picture above shows all one-sided tetrominoes with one notch except for the I-tetromino covering a square ring of size 13²-1².

Sometimes the joined sets have an odd number of notches or other conditions are not met. The table lists some possible constructions and some unsuited combinations of sets.

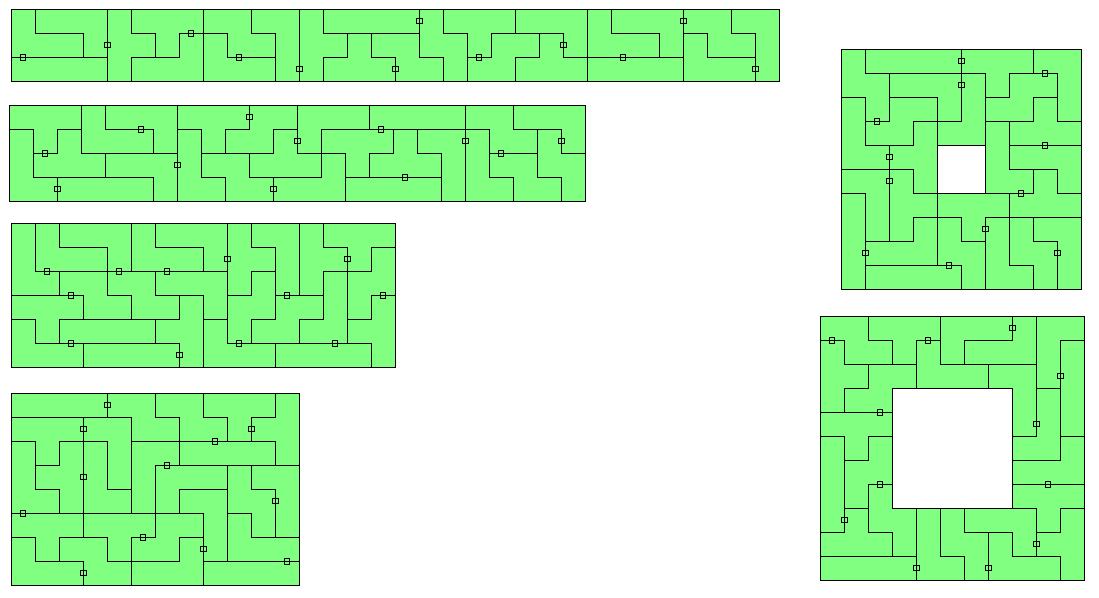
	Number of Notches	Tetromino Type					Number of Pieces	Total Area	Constructions
Two-sided Pieces	1	S	L	T	I	O
		5	10	6	3	1
		X	X	-	-	-	15	60	no rectangles odd number of notches
		X	-	X	-	-	11	44	no rectangles odd number of notches
		X	X	-	X	-	18	72	4x18, 6x12, 8x9 rectangles
		X	X	-	-	X	16	64	8x8 square, 4x16 rectangle
		X	-	X	X	-	14	56	4x14, 7x8 rectangles
		X	X	X	X	-	24	96	3x32, 4x24, 6x16, 8x12 rectangles 11²-5², 10²-2² square rings
		X	X	X	-	X	22	88	4x22, 8x11 rectangles
	2	25	45	25	15	6
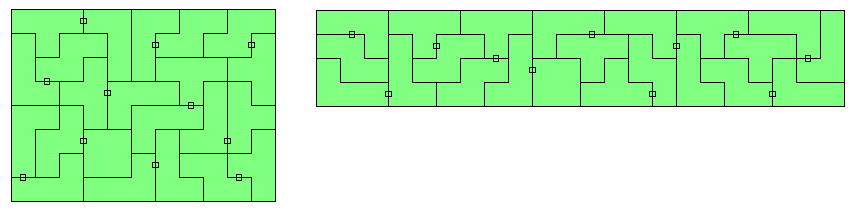
		X	X	-	-	-	70	280	no rectangles L-tetromino covering of even and odd columns or rows is unbalanced
		X	-	X	-	-	50	200	no rectangles T-tetrominoes are unbalanced under checkerboard coloring
	3	60	120	64	32	7
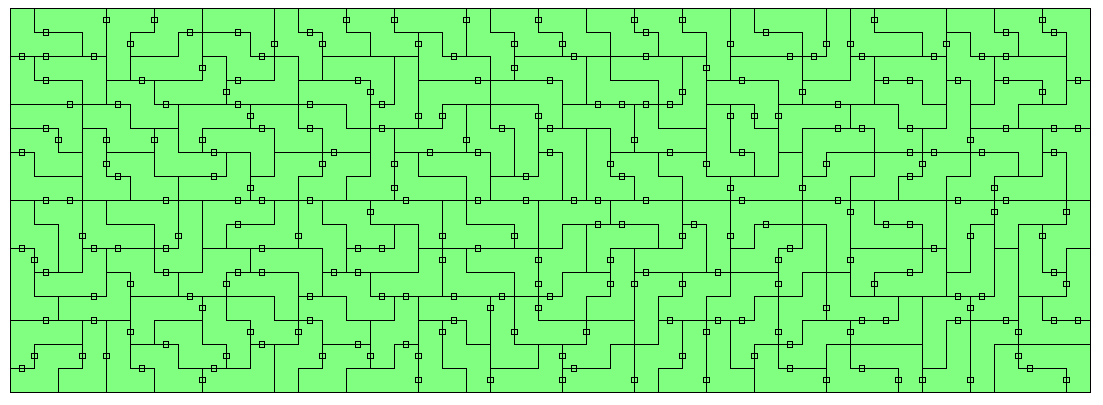
		X	X	-	-	-	180	720	16x45 rectangle
		X	X	X	-	-	244	976	16x61 rectangle
		X	X	X	X	-	276	1104	16x69 rectangle
One-sided Pieces	1	10	20	10	5	2
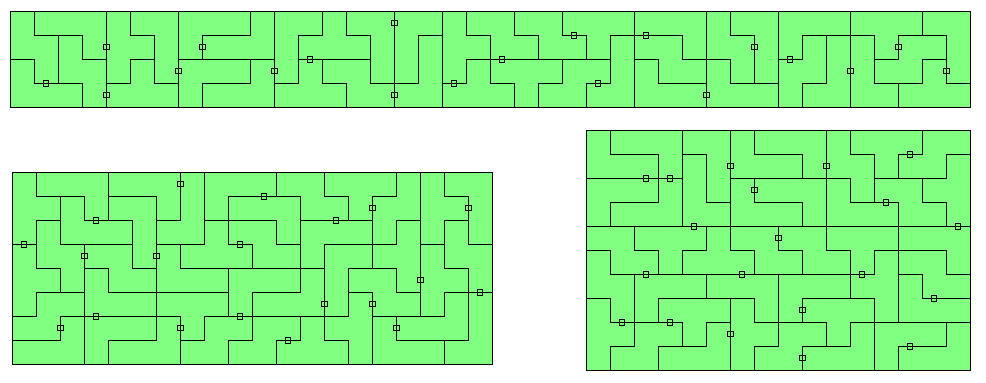
		X	X	-	-	-	30	120	8x15, 10x12 rectangles, 11²-1² square ring
		X	-	X	-	-	20	80	8x10 rectangle, 9²-1² square ring
		X	X	X	-	-	40	160	4x40, 8x20, 10x16 rectangles
		X	X	X	-	X	42	168	4x42, 6x28, 8x21, 12x14 rectangles 13²-1² square ring
	2	50	90	45	25	8
		X	X	-	-	-	140	560	8x70 rectangle
		X	-	X	-	-	95	380	no rectangles T-tetrominoes are unbalanced under checkerboard coloring
	3	120	240	120	60	14
		X	X	-	-	-	360	1440	16x90, 32x45 rectangles
		X	-	X	-	-	240	960	32x30 rectangle

There are 5 S-tetrominoes with one notch each. To get a set with an even number of notches we can add the three I-tetrominoes with one notch or the single O-tetromino with one notch. Since the number of L-tetrominoes with one notch and the number of T-tetrominoes with one notch are even, these pieces can additionally be joined.

There are 24 pieces in the union of S-,L-,T- and I-tetrominoes with one notch. The total area is 96 and allows for some rectangles and two square rings.

Using S-,L-,T- and O-tetrominoes you can cover a 8x11 or a 4x22 rectangle.

Smaller sets without L-tetrominoes or without T-tetrominoes can also fill rectangles as mentioned in the table above. It seems to be possible to solve these problems without computer, but the solutions are here. An exhaustive search showed that the set of S-,T- and O-tetrominoes with one notch can't cover a rectangle.

There are 60 S-tetrominoes with 3 notches. If we add the 120 L-tetrominoes with 3 notches, we can cover a 16x45 rectangle.

With a 16x16 square made of T-Tetrominoes with 3 notches or a 8x16 rectangle made of I-tetrominoes with 3 notches this construction can easily be extended

Let's have a look at the one-sided tetrominoes with one notch. The 5 I-tetrominoes aren't useful, because the total number of notches must be even. The total area of all other pieces including the S-tetrominoes is 168 and the pieces can cover rectangles of size 4x42, 6x28, 8x21 and 12x14

A 11²-1² square ring is shown in the title.

We can omit the two O-tetrominoes and construct rectangles of size 4x40, 8x20,10x16.

Sets of one-sided S- and L-tetrominoes with one notch or one-sided S- and T-tetromioes with one notch can also cover rectangles or a square ring. The solutions are here.

We have 50 one-sided S-tetrominoes with two notches. Since the 45 T-tetrominoes with two notches are unbalanced under checkerboard coloring, I added the 90 one-sided L-tetrominoes with two notches to the set and constructed a 8x70 rectangle.

Other constructions should be possible, too.

For each two-sided S- or L-tetromino there are two one-sided pieces, because these pieces don't have a reflection axis. Therefore we can mirror the construction for a 16x45 rectangle made of two-sided pieces to get solutions for a 16x90 and a 32x45 rectangle made of one-sided pieces. A solution with all S-Tetrominoes being connected is possible, too.

A construction with the one-sided S- and T-tetrominoes seems to be rather difficult, because the T-tetrominoes are symmetric and the reflection method can't be used. At the 1st of April I found a solution. First the whole set of two-sided S-tetrominoes together with some T-tetrominoes were packed in two 18x8 rectangles. These rectangles could be mirrored to get a 18x32 rectangle. The remaining one-sided T-tetrominoes were used to fill a small 16x8 rectangles several times. During this procedure it was counted how often each pieces was used. Then pieces with high counts mainly those with straight long sides could be saved for the end game. A 32x4 rectangle with a straight border at the right part was filled with low frequency pieces and at last with many good pieces left the remaining rectangle of size 32x8 was solved.

Back

Home