Polysticks in the Hexagonal Grid

2014/07/13
You can join line segments in the hexagonal grid to get objects made of sticks with angles of 120 degree between each other.
David Goodger called these pieces Polytwigs
and examined different sets of these pieces with up to six sticks. His
wonderful site shows a lot of constructions and I was happy to contribute a few of them.
Furthermore I was interested to make physical realizations of the sets with different materials and additionally explored
the set of pieces with seven sticks.
| Number of Sticks |
Number of Two-sided Pieces |
Material |
Number of One-sided Pieces |
Material |
| 5 |
12 |
wood |
19 |
beads |
| 6 |
27 |
acryl |
49 |
hard foam |
| 7 |
78 |
plywood |
143 |
magnetic foil |
Two-sided Pentasticks in the Hexagonal Grid
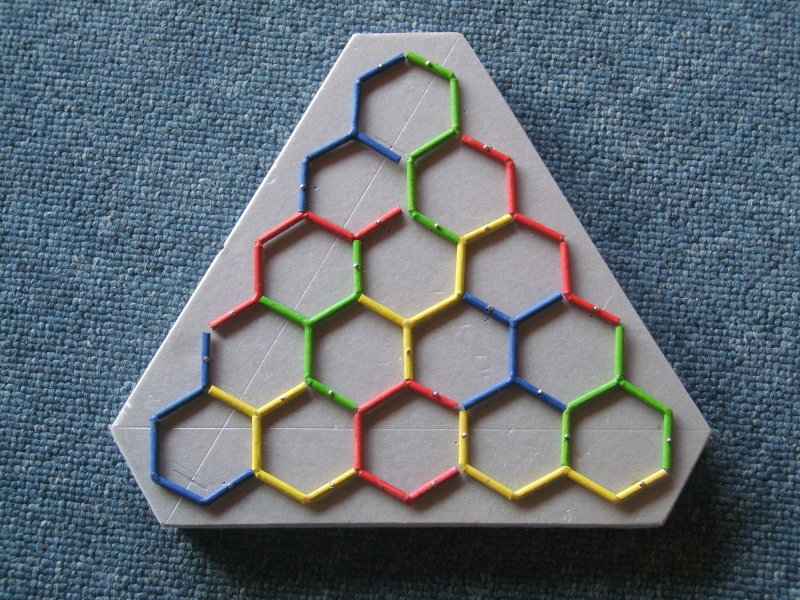
I cut wooden sticks with a diameter of 3mm and joined the sticks with fixing plastic, which hardens in seconds with a LED lamp.
Then I colored the pieces and drilled small holes through one stick. Now you can try to solve some figures
or you can fix your construction with little needles on a foam board.
One-sided Pentasticks in the Hexagonal Grid

For the one-sided pieces with five sticks I used another approach. I arranged same colored beads due to the shape
of the pieces and joined them by ironing their back side. Unfortunately the connections are very weak and the pieces
cannot be used to solve puzzles, because the often fall apart. But to display a special construction they can be
fixed with little needles on a foam board.
Two-sided Hexasticks in the Hexagonal Grid
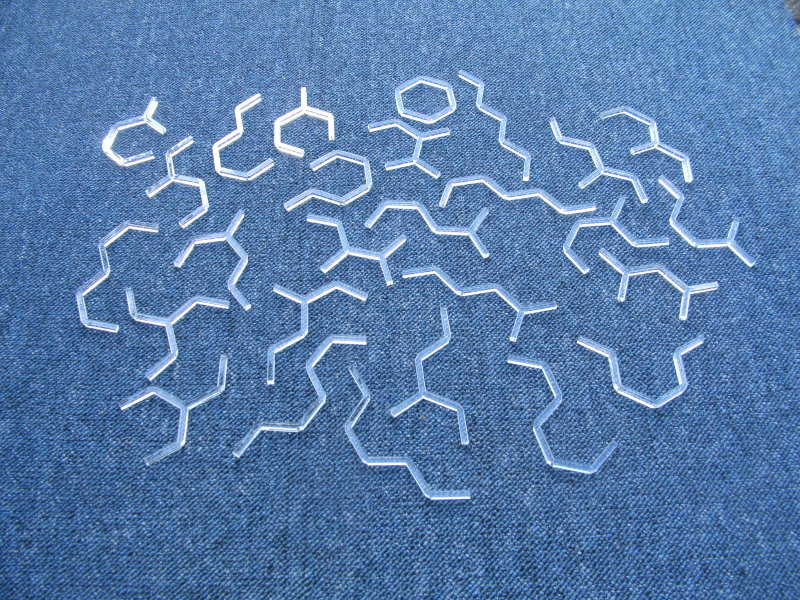
For the larger pieces with six sticks sturdy connections and high precision of the shapes are needed. Therefore I wrote a procedure to output the pieces as SVG file,
which was used to laser cut them from transparent acrylic. One construction with these pieces is already shown on David Goodgers's site.
Because the cut edges are smooth it's possible to join the pieces with two-sided adhesive film to get a construction,
which you can hang up with one thread.

Some other figures were laid down on the floor.

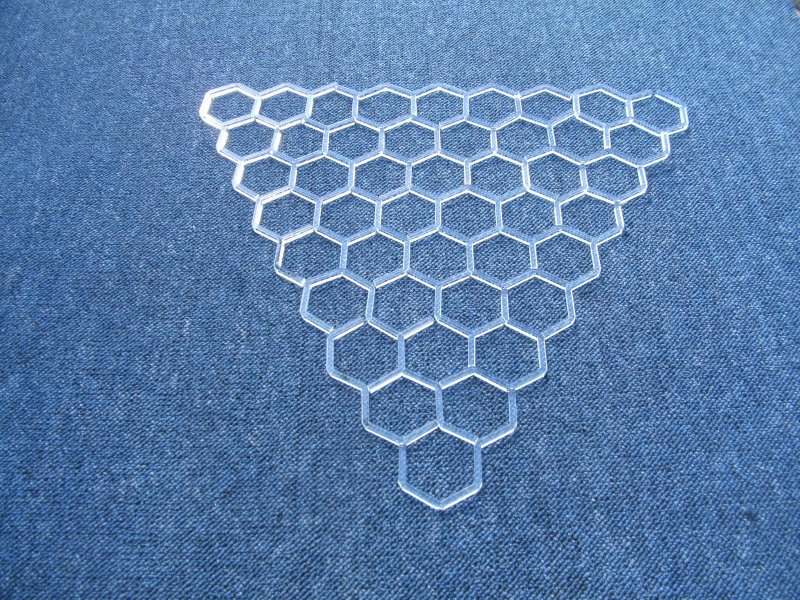
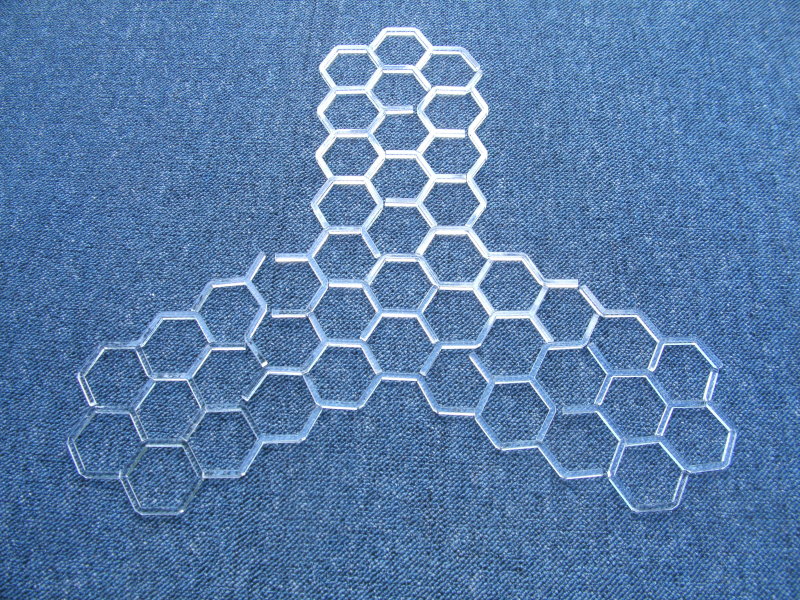
One-sided Hexasticks in the Hexagonal Grid
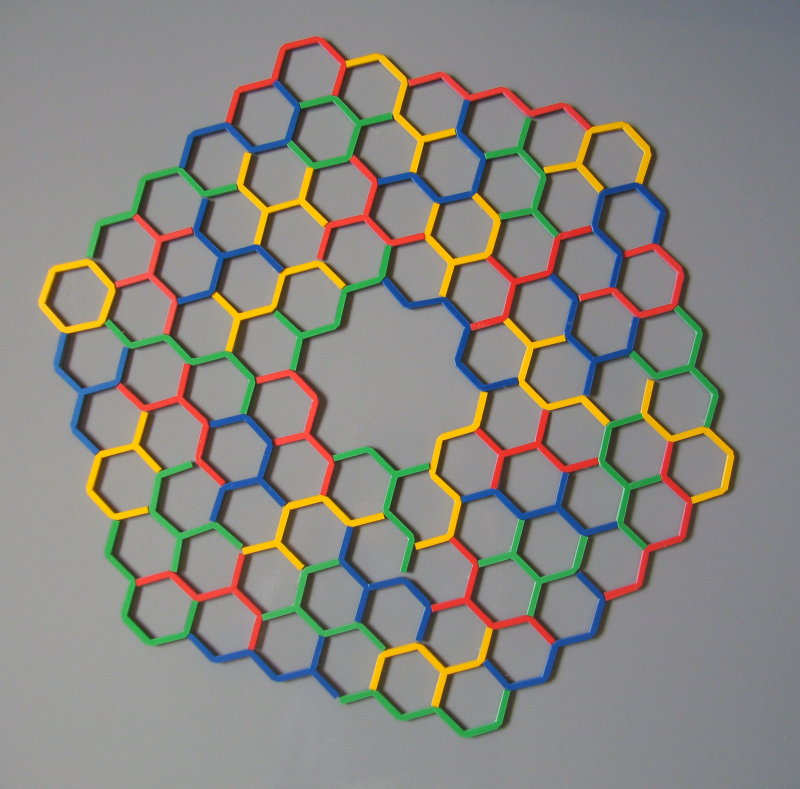
The one-sided pieces with six sticks are made from hard foam board with little magnets on the back side.
After the SVG-file of the pieces was printed on paper, I stick the paper on hard foam board and cut out the pieces.
I didn't like to spend the money for another laser cut. The coloring was done for this special construction. If
you choose other figures, pieces of same color probably would touch each other.
Two-sided Heptosticks in the Hexagonal Grid
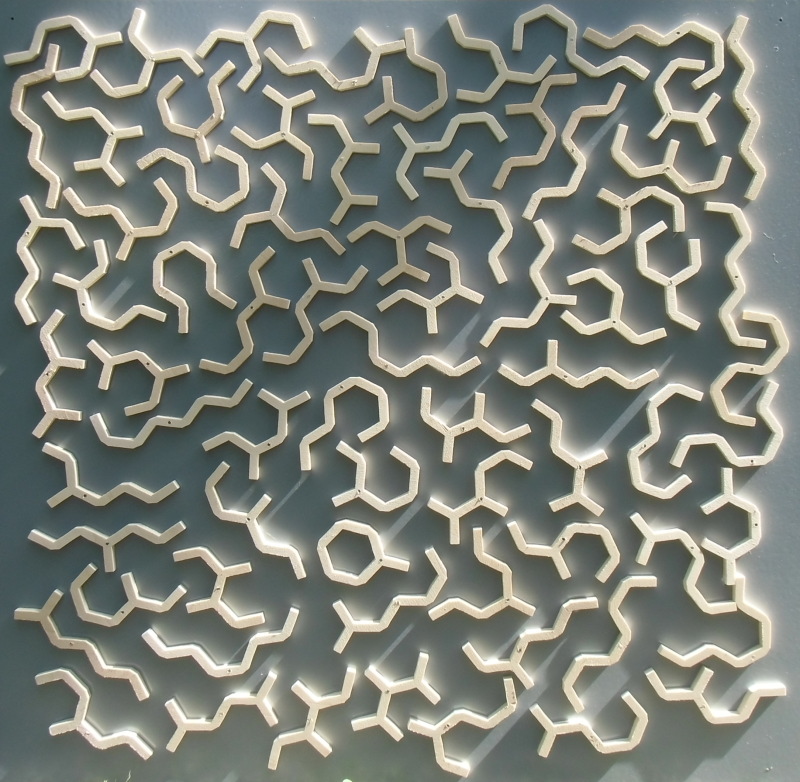
There are 78 two-sided pieces with seven sticks each. So the total number of sticks is 546 not quite enough for
a hexagon of size 8 made from 552 sticks, but omitting 6 line segments would allow for a nice symmetric figure. Unfortunately this doesn't work.
Let's have a look at the nodes of the pieces and the nodes in the figure. An odd node is an open end of a line segment or a point where three line segments meet.
Therefore odd nodes in the figure need at least one odd node of the pieces to get covered. The above mentioned hexagon has 336 odd nodes or with six line
segments removed at least 324 odd nodes. Otherwise the set of the pieces can only contribute 316 nodes.
To reduce the number of odd nodes in a figure you can increase the perimeter and this way I got some figures
for the set with different symmetries.
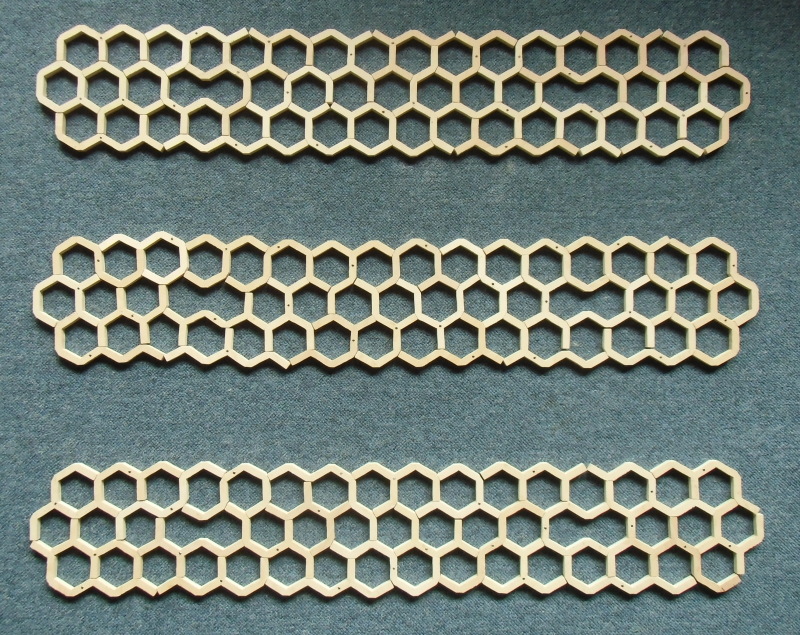
I cut the pieces from 8mm plywood and drilled holes with 3mm diameter for magnets.
Now the pieces can be put with both sides on an iron board. Therefore the first two constructions were made to fit on
existing boards. Click the pictures to see the display of the corresponding SVG-file.
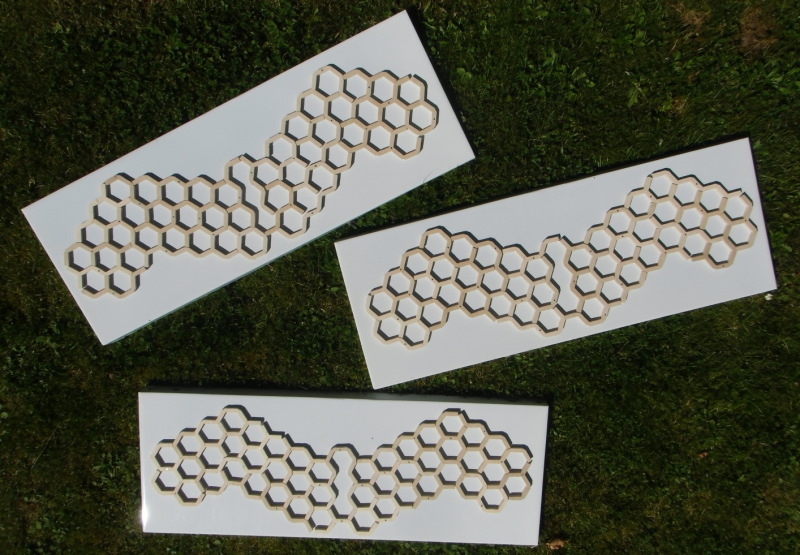
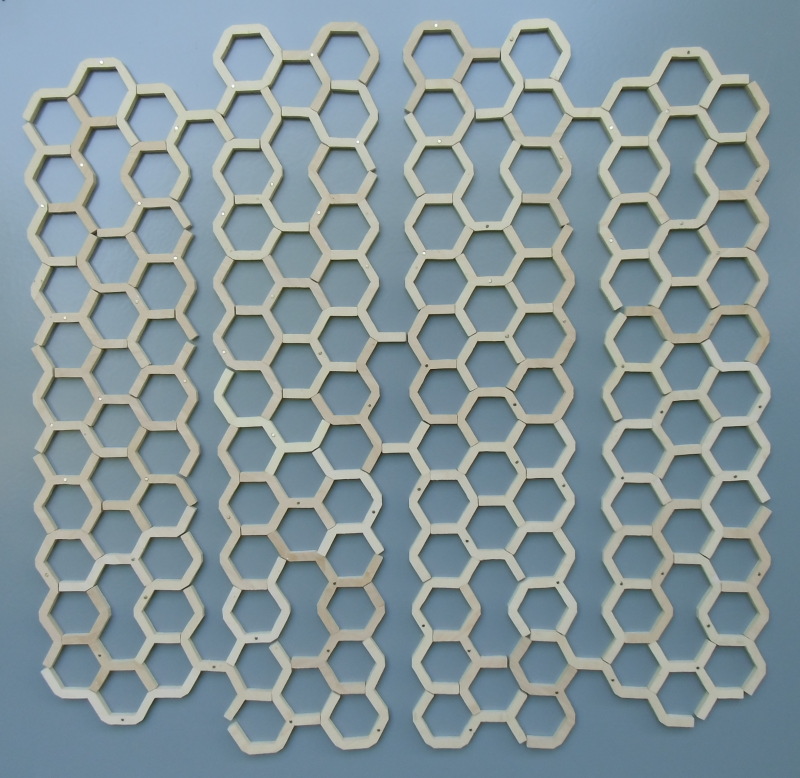
Some other figures were laid down on the floor.

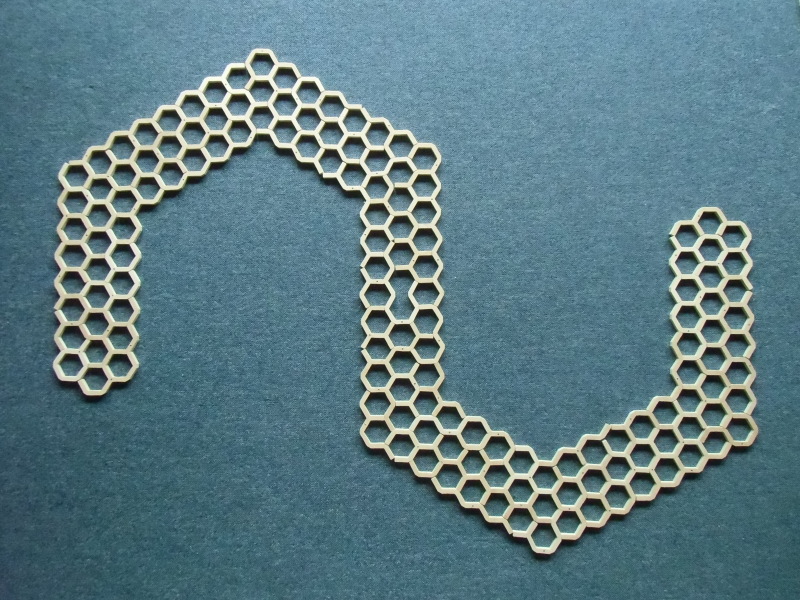
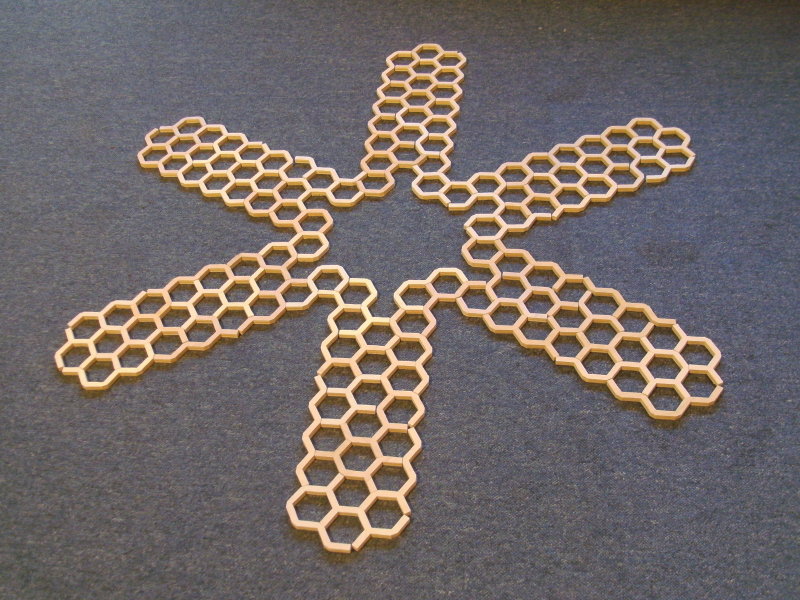
Three congruent figures with two axis of symmetry are possible, too.
One-sided Heptosticks in the Hexagonal Grid
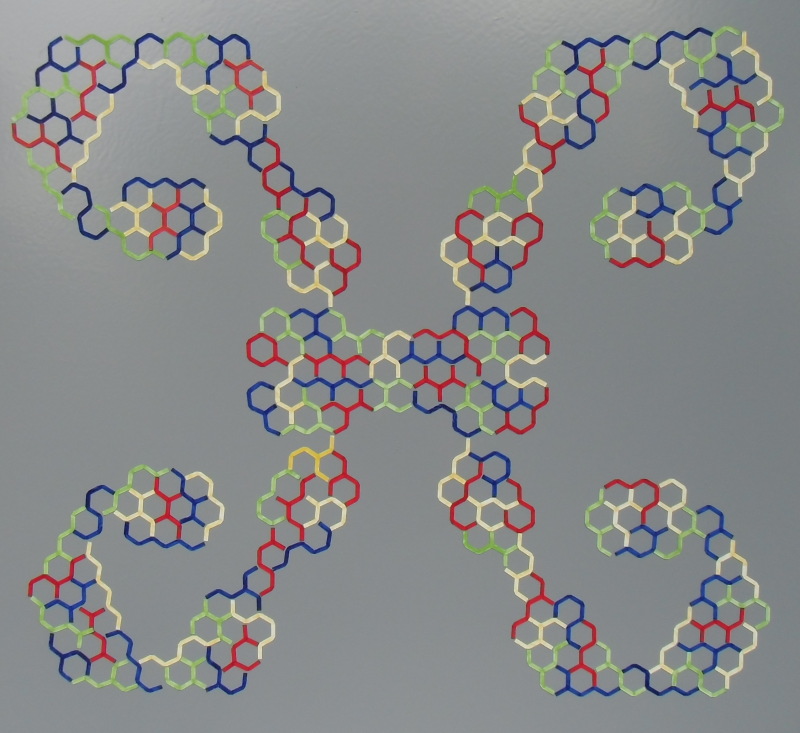
The set of the 143 one-sided pieces with seven sticks is rather hard to work with. At last I constructed the center part of a figure
with symmetric pieces and pairs of mirror pieces and covered half of the remaining area using only one piece of each remaining pair.
Reflecting this part solves the problem.
For a physical representation I took magnetic foil, which was cut by a cutting plotter using a SVG-file. Cutting plywood with a jigsaw
would have been too bothering and cutting acryl with a laser cutter would have been too expensive.
The solution for the center part was used for two other constructions, which are shown as SVG-files.


Home

















