Square Tetracubes with Pegs and Notches
Here are the 30 square-tetracubes with exactly one peg and one notch.

We can combine two 2x3x10 boxes to get 2x6x10, 4x3x10 and 2x3x20 boxes.

The 2x5x12 and 4x5x6 boxes are given by two congruent 2x5x6 boxes.

For the missing 2x4x15 box we need a special construction.
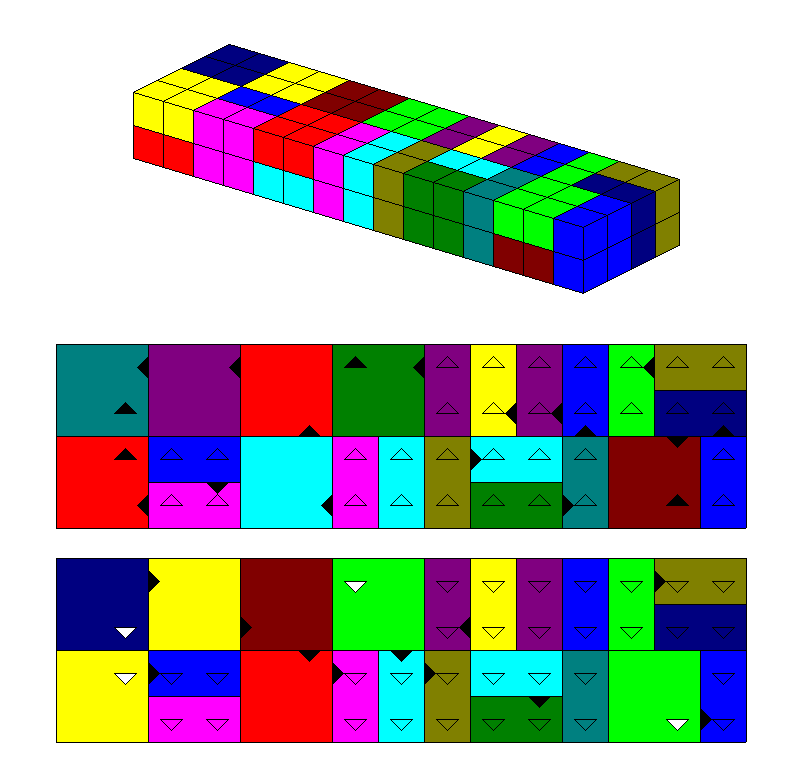
The 2x2x30 box can't be built because the pieces 1, 5, 9 and 13 would show at least with one peg or notch
at the surface of this box.
The 3x5x8 box can't be filled with square tetracubes, because the area of the 3x5 layers is odd and each piece can
only contribute 2 or 4 cubes to each layer.
There only 4 pieces with one male or one female face and the 2x2x4 box is very easy to make.


The total volume of pieces with two male or two female faces is 160 and you can construct a lot of boxes.
Two congruent boxes of size 2x4x10 or two boxes of size 2x5x8 are found very fast and can be combined to get
4x4x10, 2x8x10, 2x4x20 and 4x5x8 boxes.

Back
Home








