Drilled Tricubes
Tricubes are the best pieces to drill and to combine for constructions.
There are 8 I-tricubes with 2 holes and 12 I-tricubes with 3 holes. With the smaller set 6x4x1 and 8x3x1 rectangles are possible
but the 12x2x1 rectangle, the 2x2x6 box and the 2x3x4 box are not.

With the larger set you can get a 6x6x1 square and a 3x3x4 box but no 2x3x6 box.
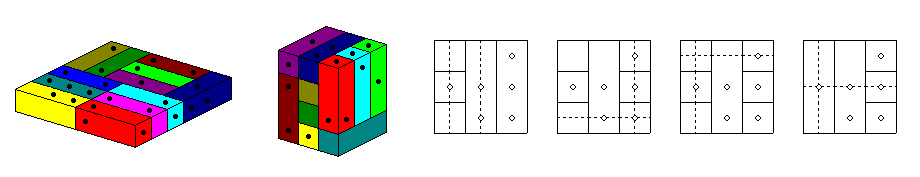
Joining all I-tricubes with 0 up to 7 holes we get 48 pieces and a total volume of 144.The set is shown on the title page,
here are three boxes and their layers.

Beside the boxes it should be possible to make 6-fold replicas of the tetrominoes with height 1 and
3-fold replicas of the tetrominoes with height 4.

With wooden pieces it looks like that and the missing replicas with exception of the square tetromino are
here.

You can also try for 3-fold replicas of octominoes with height 2.

We have 12 L-tricubes with 2 holes and we can make
3 boxes, a jagged tower and a triangle with this set.

You may ask for rectangles of size 6x6x1, 3x12x1 or 4x9x1, but these figures are impossible. The total hole length of the holes parallel
to the L-shaped faces is 21 but the total hole length of the 6x6 square must be a multiple of 6. No solution was found for the other rectangles by
an exhaustive search. There are several possibilities to select 9 out of the 12 pieces to make a cube. In this case the total hole length must be
a multiple of 3, but the condition isn't sufficient. Because this could be a nice puzzle to solve by hand, I decided to make the real pieces from wood.

If we join all L-tricubes with 0 up to 7 holes the total volume is 216, which is just enough for a 6x6x6 cube. Unfortunately I haven't got a
solution until now.
We can join the I and L-tricubes with 2 holes getting a set of 20 pieces with a total volume of 60.

With this set I produced all 2-fold replicas
of the pentominoes with height 3. The X-pentomino is shown,
the other replicas and their layers are also provided.

At last you can see four boxes of size 3x4x5, 2x3x10, 2x5x6 and 1x6x10.

Index
Home











