Cubes with 3 Colors

With 57 = 3*19 cubes you can't make a box where all edges are longer than 1. Therefore I looked
for some other figure and found a house with opposite faces same colored. The layers of the
construction are here .

To get some boxes I first discarded the three cubes with only one color and worked with the rest of the
set. With this set a 3x3x6 box is possible with opposite faces same colored,
even two 3x3x3 cubes can be made.
The layers are here . The 2x3x9 box isn't possible with this coloring,
because the two 3x9 faces contain
all cubes and must have one common color, but 9 cubes don't have one special color.
So lets look for another kind of coloring the outside faces. If we use checkerboard coloring the
reduced set isn't suitabel because of parity problems. Therefore I started with the full set of
57 cubes and left three cubes.
This way a 3x3x6 box ( see layers ) and
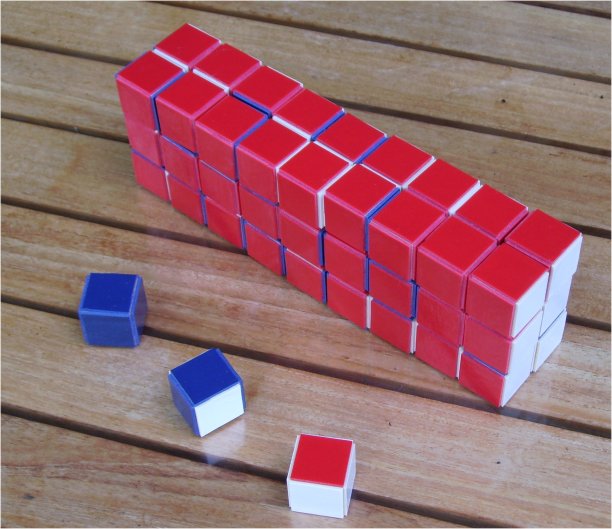
a 2x3x9 box ( see layers ) are possible.
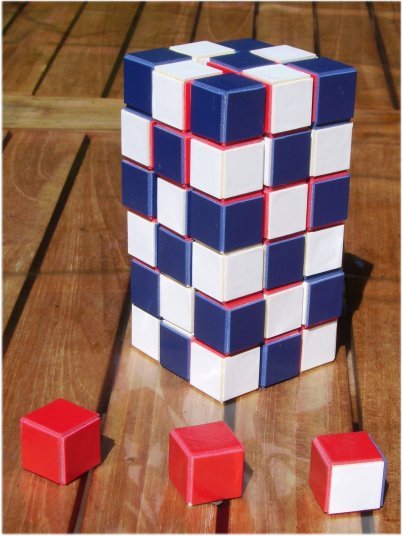
For the 3x3x6 box, you can also hide one color, if two pairs of three same colored faces exist.
( see layers )
For the 2x3x9 box a construction with uniformly colored faces is possible,
if we choose three pairs of neighbouring faces with same color. ( see layers )
Index
Home




