2 Colors, No restrictions
Without any restrictions we get 36 one-sided and 30 two-sided octagons. With these sets we can construct rectangular figures of size 6x6 and 6x5,
respectively. For other rectangles the solutions are even easier.
The above solutions can be extended by copies of themselves to cover the plane. This is shown for the 6x6 square.
2 Colors, 2 Colors on Each Piece
If the same colored pieces are discarded we have 34 one-sided and 28 two-sided pieces.
With the 34 pieces I made a jagged oval and a 2x17 rectangle.
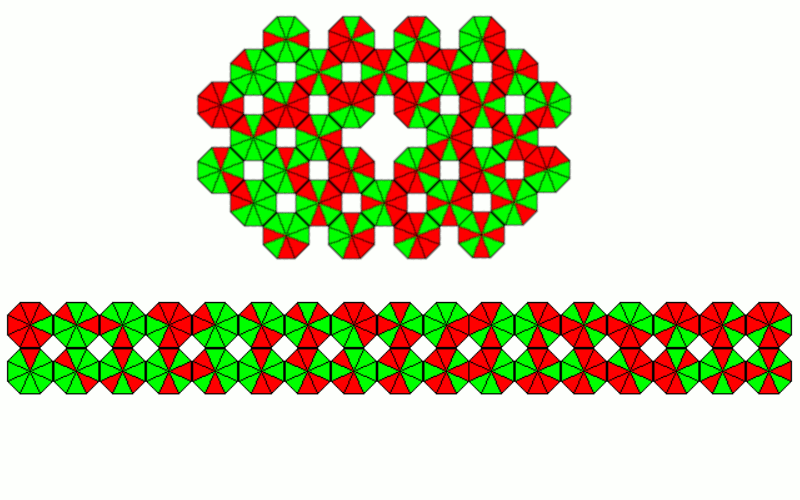
With the 28 pieces a 4x7 rectangle and a jagged rectangle is shown.
If we restrict the frequencies of colors to a fixed ratio, the sets are very small and solving patterns no problem.
2 Colors, Ratio of Color Frequencies is 2:6
If 6 sides of a piece get one color and 2 sides the other color the piece is symmetric, so that the set of one-sided pieces and the set of
two-sided pieces are identical. Here are a 2x4 rectangle and a ring of size 3.
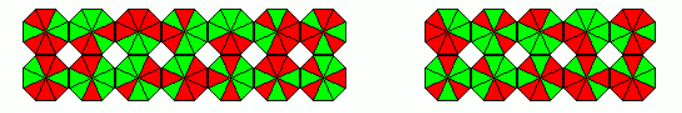
2 Colors, Ratio of Color Frequencies is 3:5
For the 3:5 color ratio we have a 2x7 rectangle using the 14 one-sided pieces and a 2x5 rectangle using the 10 two-sided pieces.
2 Colors, Ratio of Color Frequencies is 4:4
If all octagons have 4 sides of each color we get sets of 10 one-sided pieces or 8 two-sided pieces.
The 10 octagons can make a 2x5 rectangle and the 8 octagons a 2x4 rectangle or a ring of size 3.
Back
Home