0ne-sided Bridged Tetriamonds
For the definition of the pieces look at the section about
bridged polyiamonds. Here are some
construction with the real pieces printed with a 3d-printer. We have three symmetric convex polygons using all pieces.



Even if a computer solution is given you have to spot the right piece in a large set.
Therefore you should sort the pieces. In the following picture we have six subsets:
- pieces with no or one bridge
- pieces with four single triangles one of them directly connected to the three other ones
- pieces with a diamond which is connected at only one corner
- pieces with a diamond which is connected at two corners
- pieces with a chain of triangles where the center connection is X-shaped
- pieces with a chain of triangles where the center connection is M-shaped

I added a second triangle shaped piece to avoid parity problems with this set and got some more symmetric constructions.
Now the above trapezium can be changed into a triangle.

With the following diamonds or with the trapezium and the triangle 12-fold replicas
of the pieces with at most one bridge can be made.


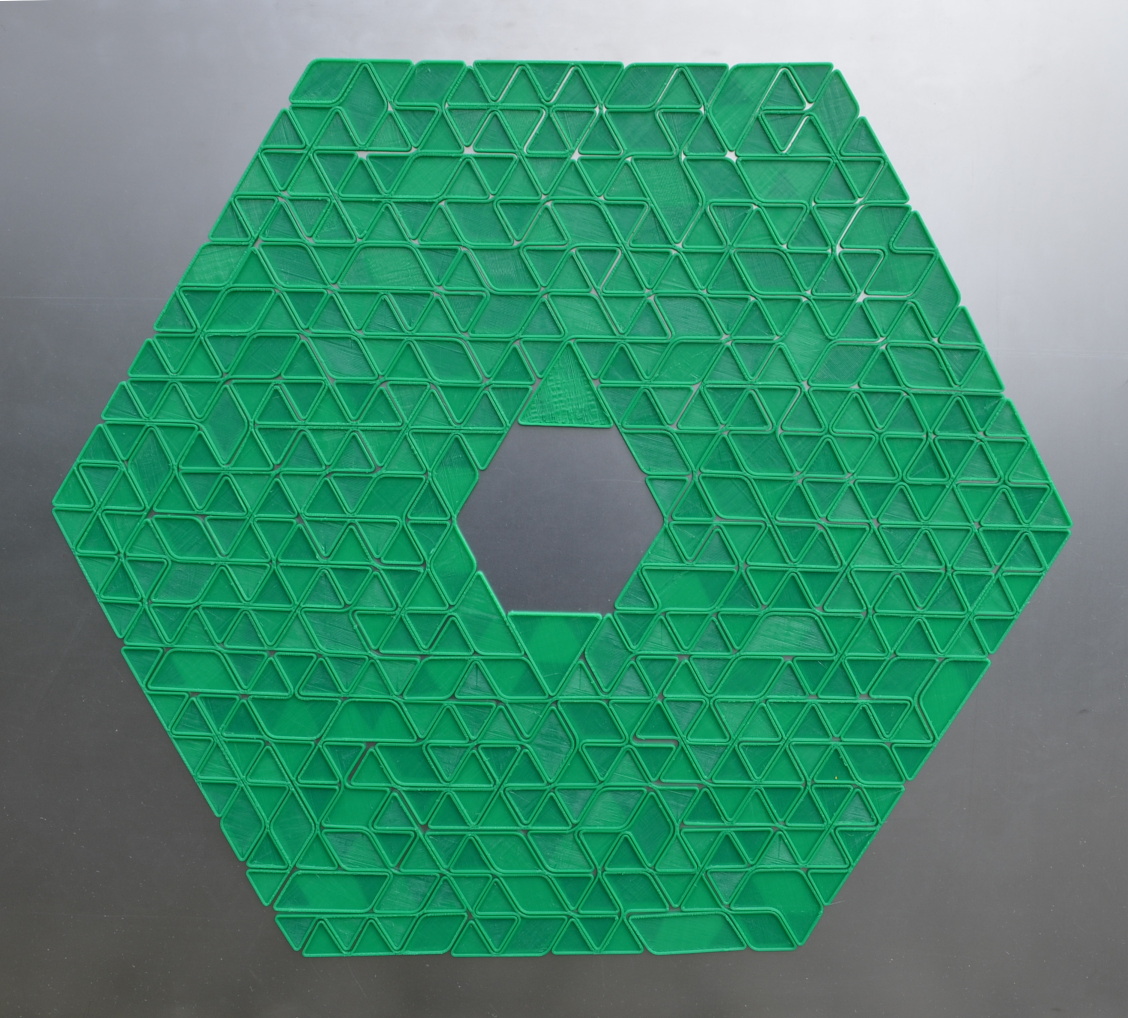
Let's take a look at the trihexes. For a 4-fold replica of such a trihex we need 72 pieces. If a pair of such
pieces is replicated the whole set can be used. Two pairs are shown and can be combined to get the enlarged
triangle hexhex or the ring hexhex.


The other combinations are also possible:
(bar-bar)
(triangle-triangle)
(arc-bar)
(arc-triangle)
With these combinations almost all hexhexes can be replicated.
Index










